SATs 2024 – The ultimate teacher guide to preparing pupils for Key Stage 2 assessment

How to prepare pupils for this year’s SATs and make sure they get the scores they deserve…

- by Teachwire

Whatever your thoughts and feelings are about SATs, and tests in general, naturally you’ll want your pupils to do as well as possible, without causing them any stress or worry along the way.
We’ve picked out a selection of resources that can help consolidate children’s learning and prepare them for SATs, without making it feel like a big, dark educational cloud is looming on the horizon…
When is SATs Week 2024?
In 2023, the SATs original schedule was changed due to King Charles’ coronation. Hopefully there will be no disruption this year, with KS2 SATs due to take place between Monday 13th May and Thursday 16th May 2024.
The timetable is as follows:
| Monday 13th May | English grammar, punctuation and spelling papers 1 and 2 |
| Tuesday 14th May | English reading |
| Wednesday 15th May | Mathematics papers 1 and 2 |
| Thursdays 16th May | Mathematics paper 3 |
It is recommended that the optional KS1 tests are administered during May 2024.
When are SATs results 2024 announced?
Results will be made available to headteachers on 11th July 2024, and passed on to parents around this time. Schools’ performances will be made public in December 2024.
SATs scaled scores 2023
Scaled scores for 2024’s SATs will be made available in July 2024. In the meantime you can look at SATs scaled scores for 2023.
KS2 English SATs resources and advice
KS2 English – SATs practice tests
You can find KS2 past papers from 2019-2023 on the government website.

Make sure Year 6 pupils are prepared for SATs with Plazoom’s KS2 SATs Support collection. It contains reading test practice packs, ten SPaG practice papers, writing evidence activities and fun ‘Revision Blasters’ that are linked to content domains for grammar.
Each Revision Blaster grammar pack includes a recap section for reteaching and a practise section with SATS style questions. They’re perfect for SPaG revision in Year 6.
The ten practice SPaG papers feature 12 questions which follow the same format as paper 1 of the English grammar, punctuation and spelling test. All content domains are covered across the collection at least twice.

These free grammar, punctuation and spelling practice test papers from STP Books are modelled on actual SATs exams and come with complete answers and marking guidelines.
Year 6 SATs spelling practice
If you want to focus on helping children learn and master spelling patterns, rules and exceptions, visit the Get Spelling Sorted collection on Plazoom. It’s a great repository of revision games and activities.
Use this free top 40 words spelling sheet download in Year 6 to practise these common and sometimes tricky words.
Browse more Year 5 and 6 spelling list resources.
Year 6 SATs writing evidence

If you need to gather more independent writing examples to assess Year 6 pupils against the writing Teacher Assessment Framework, use these Year 6 SATs Writing Evidence packs from Plazoom. They’re specially designed to get children producing authentic, independent writing.
Each set of activities is linked to foundation subjects, providing opportunities for cross-curricular writing. For example, write a non-chronological report about bridges, a persuasive report about keeping healthy or a diary recount of an historical event.
Year 6 SATs comprehension

This free sample practice paper from CGP contains a reading text extract, questions and pupil-friendly answers.

If you’re looking for more Year 6 SATs reading comprehension practice PDFs, try these options from Plazoom.
Each of the three packs has been carefully designed to follow a similar format to the SATs. This means your pupils can become more familiar with their layout and improve their confidence.
Use them as practice tests or use them as part of group or whole-class reading sessions. You could also use them as smaller comprehension activities where pupils read the text and complete the questions.
Choose from Set A, Set B or Set C.
Reading paper revision tips
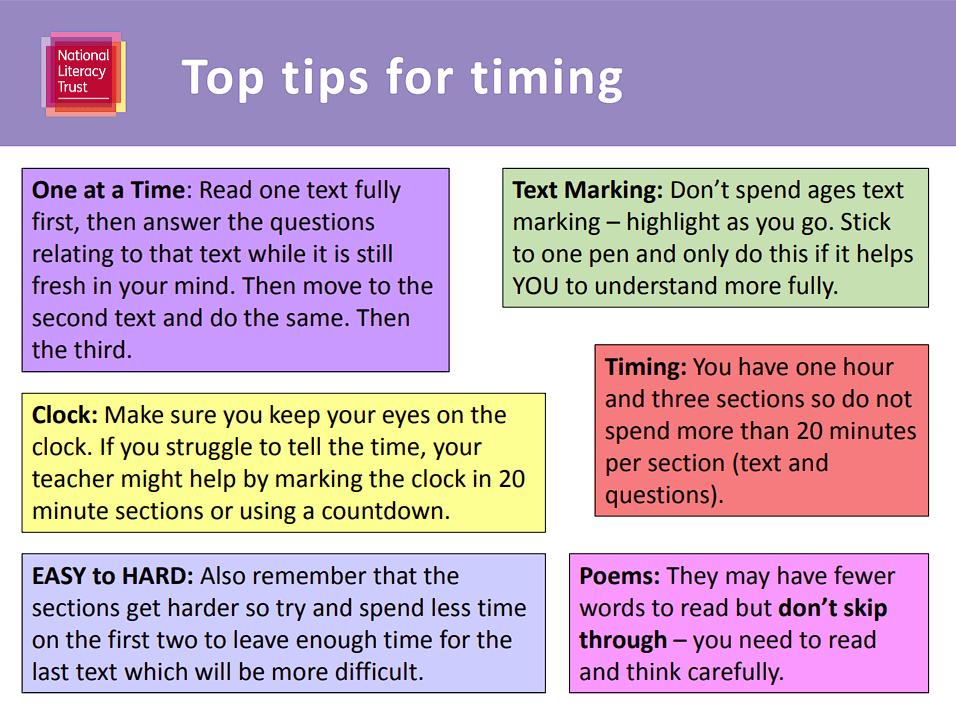
Use this revision guide PowerPoint from the National Literacy Trust to remind pupils of the key aspects of the reading paper before the big day.
English SATs preparation Year 6 advice
Make sure children get the scores they deserve with these last-minute and not-so-last-minute SATS checks from Shareen Wilkinson…

Step 1 – Be aware of the NC expectations for Y6
It can be tempting to enter revision mode right from the start of Y6, but don’t forget there are some topics that won’t yet have been taught.
There are, of course, key areas that will need to be revised through the year. However, the following list picks out the parts of grammar that are unique to your pupils’ final year at primary school:
- Semi-colon and colon
- Bullet points
- Subject and object
- Synonym and antonym
- Ellipsis
- Hyphen
- Active and passive
Step 2 – Review previous year groups
The grammar and reading papers contain many questions about topics not first taught in Y6.
Some Y3 and Y4 teachers openly admit to avoiding grammar areas they are not confident about teaching. It’s not fair to leave it all to Y6, but we all know this sometimes happens!
“It’s not fair to leave it all to Y6, but we all know this sometimes happens!”
Revise content from previous year groups throughout the year and save explicity teaching test techniques until later on in the spring term.
For example, when teaching semi colons and colons, also revise what makes a sentence. Knowledge of an independent clause is needed to understand when and how to use a semi colon.
Step 3 – Drip in test-style questions
Repetition is vital. The more pupils encounter test-style questions in a practical and fun context, the less fazed they will be when it comes to the actual test.
In practice, this simply means that when completing a reading lesson, adjust your questioning.
For example, instead of saying, ‘Why does the character…’, this could be tweaked to ‘According to the text, what impression do you get of the character…’.
Step 4 – Keep reading for pleasure and information
The way to develop vocabulary and be exposed to grammar in context is to read a wide range of fiction and non-fiction texts.
This broadens pupils’ experiences and ensures they have a rich vocabulary and knowledge to prepare for the demands of the tests.

Step 5 – Explicitly teach test techniques
Teaching test techniques is sometimes addressed by giving pupils endless past papers to complete. This is nearly always (in my experience) a useless and boring activity.
There is a place for pupils to experience what a whole paper looks like (explored in Step 9) but there is a benefit to explicitly teaching skills.
For example, pupils need to be clear about the question stems used in the reading paper. They also need to have an in-depth understanding of the vocabulary.
An ‘impression’ is normally an inference question, so pupils need to find clues in the text to support their answer.
When answering fact and opinion questions, pupils need to think about whether a statement is true or false. In addition, is it what someone thinks or feels and is it stated or implied?
This level of explicit teaching will support pupils’ understanding of reading test vocabulary.
Reading vocabulary to teach
- According to…
- How can you tell…
- Find and copy…
- Give two impressions…
- Fact and opinion
- Give the best summary…
- What does this suggest…
- Using evidence from the text to support your answer…
Step 6 – Avoid errors in the grammar test
One important factor concerning the grammar test is that there are very definite rules about how to answer the questions.
Answers need to be clear and unambiguous. Prefixes and suffixes, verbs, contractions and plurals need to be spelt accurately and the correct number of boxes should to be ticked.
It is so frustrating when pupils miss a standard because of small errors, but we’ve all seen it.
Step 7 – Use fun and interactive revision strategies
Who says that revision has to be dull? I often hear this and it doesn’t reflect my experience at all.
Teaching inference skills can easily be covered by exploring the thoughts and feelings of characters in pop songs.
Grammar should be explored in context. For instance, try creating wanted posters for word classes where pupils need to describe their function.
The best way to consolidate learning is to get pupils to teach other pupils in the class. Give them an area of focus and let them create a poster or worksheet that they will present to the rest of the class.
Being able to fully explain a concept to someone else shows you have really internalised the knowledge.
Step 8 – Involve families where possible
Significantly, I always hear that some families are reluctant to attend information sessions. One of the key ways to get parents and families involved is through – you’ve guessed it – food!
In the spring term, entice your families with a SATs information event that includes the important stuff – like when the tests are, what they include and how parents can help at home. Just don’t forget the biscuits and cake!

Step 9 – Give pupils experience of the test papers
Whilst it’s important to continue to teach and to drip test-style questions throughout Y6, pupils still need to be aware of the timings. This is especially true for the reading paper where they have 60 minutes to read through and answer questions for three or four texts.
Rapid retrieval is needed for this. Just try to keep test experience to a minimum.
Step 10 – Work on whole-school strategies
Having taught my own Y6 classes for ten years and been a booster group teacher, I have seen the benefits of involving and training the whole school.
The more all teachers see the test papers, have CPD opportunities to increase their subject knowledge, and the more senior leaders promote a whole-school responsibility for SATs, the higher the likelihood of success.
Even more English SATs preparation ideas

1. Tackling tough words
Teaching vocabulary skills in context is essential. Whilst we cannot predict the words that will appear on the test, we can give pupils quick strategies that will support them, whatever they encounter.
For example, take a classical text and replace certain words with nonsense words. Can the children think of plausible substitutions for your replacements? (I sometimes add capital letters to nonsense words to indicate they might be proper nouns.)
Pupils should be able to deduce what each word might be from the context.
2. Speed searches
Maintain a focus on scanning skills under timed conditions. Quick-fire activities, such as Where’s Wally or spot the difference, are perfect for continuing to develop retrieval skills before they are applied to more complex texts.
3. It’s not what ‘you’ think
It is crucial for pupils to recognise that all answers will be based on the test and not their own views.
Questions that contain the word ‘you’ are somewhat misleading. For example, ‘How can you tell…?’ ‘Give two impressions this gives you.’ Reinforce that evidence is found in the text.
4. Don’t repeat the question
This might seem like an obvious point, but it is crucial that pupils do not repeat the question stem in their answer – but rather explain it.
For example, if the question is ‘Why were the dodos curious and unafraid?’, it’s not unusual in instances like this for children to write something along the lines of, “Because they were unafraid”. This means they’ll miss out on a mark.
To avoid this, work on using synonyms and explaining answers.

5. Perfect punctuation
Accuracy is extremely important. The punctuation of direct speech will only be creditworthy if the closing punctuation is placed inside the final inverted commas.
In previous tests pupils have lost marks for inconsistencies such as mixed use of inverted commas (eg ‘ and “).
6. Read questions closely
‘Write a sentence using the word ‘point’ as a verb. Do not change the word.’
In questions like this, make sure children follow the instruction closely.
Pupils have lost marks in the past for adding a suffix (eg ‘pointed’ or ‘pointing’). They also lost marks for not starting with a capital letter and ending with appropriate punctuation.
7. Watch for stray capitals
If pupils are asked to write a sentence containing an adverb they may be penalised if they spell the adverb incorrectly or if they start the adverb with a capital letter in the middle of a sentence.
Don’t let your pupils miss out due to simple errors.
8. Avoid getting caught out
Prefixes, suffixes, verb forms and plurals must be spelt correctly.
One question in the 2016 grammar paper required pupils to change the word ‘caught’ to the present tense. The answer is ‘catch’, but even though pupils knew the answer, they lost marks for spelling ‘catch’ incorrectly.
Again, spelling does count within the grammar test – even though there is a separate spelling paper.
KS2 maths SATs resources and advice
The KS2 maths SATs test is split into the three papers – and it’s not easy to predict which topics will come up in each one.
Area and perimeter won’t show up in paper 1 (arithmetic) as this is purely a calculation paper. On paper 1, fractions questions will always involve fractions of amounts or multiplying or dividing fractions.
Papers 2 and 3 usually have questions in context. Measures, statistics and shape and space will only come up on these papers.
You can view a list of what can potentially be included in the papers on a government document called Mathematics Test Framework. This lists all the areas that could be tested – but they aren’t all included every year.
Free maths workbook
This free KS2 Maths Study Book from Collins features clear and accessible explanations, quick tests, practice questions and more.
Also available from Collins is this free set of KS2 maths flashcards.
Maths SATs questions
Fractions SATs questions
Using these free fractions SATs questions from experienced maths teacher David Morse will help to test and extend students’ understanding, as well as helping them to prepare for SATs.
You can print the eight-page PDF and turn it into an A4 or A5 booklet. You can also display the solutions on your whiteboard, making feedback more efficient.
The download contains questions about fractions of a shape and identifying how much of a shape has been shaded. It’s perfect for using in class or as homework.
Fraction arithmetic questions
David Morse has also created this free fraction arithmetic questions pack for KS2 SATs practice. It’s a 12-page PDF featuring 32 questions for pupils to have a go at.
Equivalent fractions Year 6 questions
These free printable equivalent fractions Year 6 questions have fully-worked solutions which can be displayed on a whiteboard.
More KS2 maths worksheets
Maths Blog has a whole host of printable KS2 maths booster worksheets that pupils can print off at school or at home. There are 14 PDFs for number-related questions, three for shape and four each for time and graphs.

Head over to Maths Made Easy to find more KS2 SATs practice papers. There’s two for arithmetic and four for reasoning to try.
SATs questions linked to contents domain
Headteacher Mr T has created a free download laying out Year 6 SATs questions linked to the contents domain.
Year 6 maths revision tips
Use pictorial representations to help Y6 pupils prepare

Here, sadly missed White Rose Maths senior primary maths specialist Beth Smith explains why giving pupils visual tools will help them make clear mathematical links come test time…
In the run-up to Y6 SATs, we’re faced with not only recapping previous years’ learning but also teaching the new Y6 content of the curriculum.
In order to ensure SATs don’t turn into a memory test, we need to make clear links between new learning and prior knowledge so children can build on their understanding, rather than starting afresh with every new concept.
Equal groups
One of the most important concepts that children need to understand is the use of equal groups throughout the curriculum.
In KS1, children are introduced to equal groups within multiplication and division. This starts with a basic understanding that 2 × 3 is two equal groups of three (or three equal groups of two) before deepening their understanding by comparing equal groups.
Take this example from the 2016 KS1 SATs:
Complete the number sentence below.
3 x 8 = 2 x __
Using a bar model, children can visualise the equal parts in order to help them solve the problem.
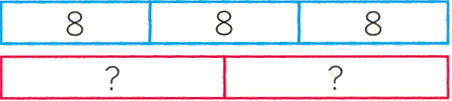
Drawing the bar model supports us to decide which calculation to use. Here, we need to find out the total of the each bar. If we multiply 3 by 8, we find the total of the bar is 24.
Once we have found the total, we can see that as the bars are equal. The second bar will also be worth 24 but this time it is divided into two equal parts.
Therefore, to find the missing information, we need to divide 24 by 2 to find our missing box is 12.
The use of the bar model for this question helps to support children to see how 24 is split into three equal parts on the top bar and into two equal parts on the bottom bar.
Using this approach in KS2
Moving into KS2 you can start to explore factors, fractions or percentages of 24.
All would involve dividing 24 into equal parts. Using a consistent pictorial method will help children see the links between the concepts.
Here’s a question from the 2017 KS2 reasoning paper.
3 pineapples cost the same as 2 mangoes. One mango costs £1.35. How much does one pineapple cost?
Again, let’s use a bar model to visualise the problem:
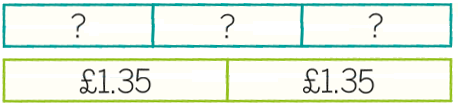
Here we can see that we need to find two equal groups of £1.35 to make £2.70 and then we need to divide £2.70 between three equal groups to find that one pineapple would cost 90p.
Interestingly, if we look at the bar models we have used for the KS1 and the KS2 question, they are pretty much identical. The only difference is the numbers used and the calculations required. The structure of the problem is the same.
Representing your thinking
In order to support children to tackle problems like this, we need to encourage pupils to represent their thinking through pictorial representations.
As teachers, we need to model and explain the clear links between questions. Another concept that builds upon children’s prior understanding of equal groups is ratio. Take this question for example:
Lucy and Jemima share £30 in the ratio 2:3. How much money does Lucy receive?
Here is a worked example on a bar model:
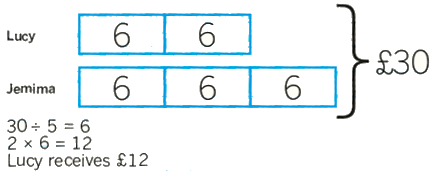
The bar model supports the procedural understanding of calculating ratio by dividing the amount by the total number of equal parts.
We can also stretch children’s thinking to answer questions such as ‘How much more money does Jemima have?’ or ‘Lucy and Jemima share some money in the ratio 2:3. If Lucy has £24, how much money do they have altogether?’
Once again, within ratio, the understanding of equal parts is essential to children’s understanding.
If we can help children to understand equal groups and parts clearly and help them to use bar models to represent their thinking, we can give all children the tools to tackle more complex problems.
Using previous SATs questions to help pupils consider links and patterns

There’s more advice here from the brilliant Beth Smith about teaching problem-solving strategies…
In the EEF’s research into improving mathematics in KS2 and KS3 (2018), one of its eight recommendations was to teach pupils strategies for solving problems.
It then provided the following suggestions:
• If pupils lack a well-rehearsed and readily available method to solve a problem, they need to draw on problem-solving strategies to make sense of the unfamiliar situation.
• Select problem-solving tasks for which pupils do not have ready-made solutions.
• Teach them to use and compare different approaches.
• Show them how to interrogate and use their existing knowledge to solve problems.
• Use worked examples to enable pupils to analyse the use of different strategies.
• Require pupils to monitor, reflect on, and communicate their problem solving.
Let’s consider some of these suggestions in relation to questions taken from SATs and White Rose Maths assessments.
Bar modelling
Firstly, bar modelling can be a brilliant tool to help children make sense of the information they are given.
Take this question from the 2018 SATs:
Amina is making designs with two different shapes. She gives each shape a value.
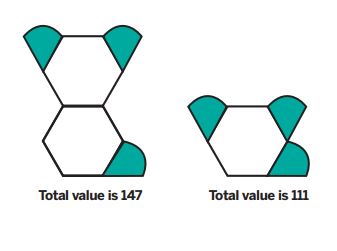
Calculate the value of each shape.
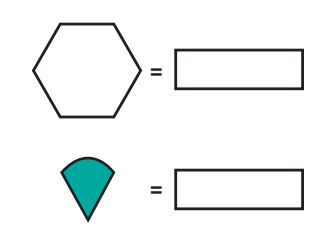
Encourage children to use a bar model to draw out what they know.
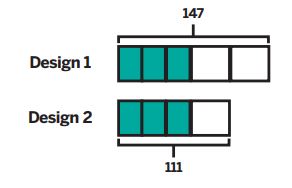
Notice the way the different colours of shapes are grouped together. This helps children to see what is the same and what is different about the bars.
Once the starting bar model has been drawn, children can start to consider what else they can find out.
The first step in this model is to consider what the difference between the designs is and therefore what the value of the larger shape is.
The annotated bar model below shows how this can be modelled:
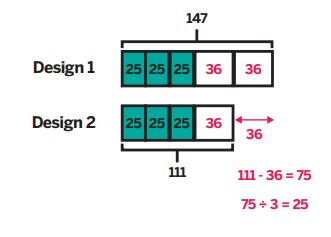
Introduce a similar problem
An important aspect of teaching problem-solving is to then provide children with another problem with a similar structure to practise their skills.
Providing the same problem with different numbers takes the problem-solving elements away and moves the question more towards fluency.
However, another problem with a similar structure but a different context allows children to refine their thinking.
Take this question from the Y6 White Rose Maths spring assessment:
The mass of a box containing 6 tins of beans is 7.5kg. When 2 tins of beans are removed, the mass of the box is 5.1kg. What is the mass of one tin of beans?
The situation is completely different. However, when placed into a bar model children can use similar skills to solve the problem.
Possibility of errors
Next, let’s consider how we can use problem-solving questions to support children to apply their knowledge and skills.
Many questions require children to calculate both mentally and through written methods, practising the skills they have acquired.
In the question below, children add and subtract to find change in a money problem:
John buys one toy car (£1.49) and one pack of stickers (£1.64). How much change does John get?
Most commonly, children will use the following method:
£1.49 + £1.64 = £3.13 £10 – £3.13 = £6.87
Some pupils will be able to attempt this mentally. However, others will rely on written methods and this can bring the possibility of errors, especially when calculating £10.00 – £3.13. Children have to complete multiple exchanges, which may lead to errors.
Constant difference
A second method, shown below, addresses this issue head on.
When subtracting, we can apply the idea of constant difference. If we add or subtract the same amount to both numbers in the subtraction, the difference will remain the same.
Subtracting one penny off both amounts (£10 and £3.13) leads to a much easier subtraction without any exchanging.
£1.49 + £1.64 = £3.13 £9.99 – £3.12 = £6.87
Consider how this method can be applied to the following questions:
Ken is playing a game. He has 4,289 points. Then he scores another 355 points. Ken’s target is 6,000 points. How many more points does Ken need to reach his target? (2019 KS2 SATs)
Morgan is running a 10 kilometre race. So far, she has run 1,340 metres. How far does she have left to run? (White Rose Maths Y6 summer)
Comparing different approaches
Finally, let’s consider how we can use and compare different approaches.
The below question from the 2019 SATs test is, on the surface, a problem requiring a number of steps involving multiplication and addition:
Layla makes jewellery to sell at a school fair. Each bracelet has 53 beads. She makes 68 bracelets. Each necklace has 105 beads. She makes 34 necklaces. How many beads does Layla use altogether?
Many children would approach this problem by using the numbers they are given and calculating in three steps:
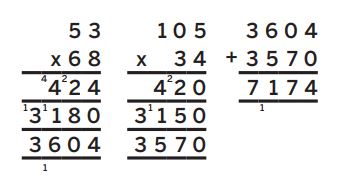
The second method, below, shows a link between the calculations.
If we notice the relationship between 34 and 68 in the question, we can use that to help us with our calculations.
Remember, when multiplying, if we half one number and double the other number, the product remains the same:
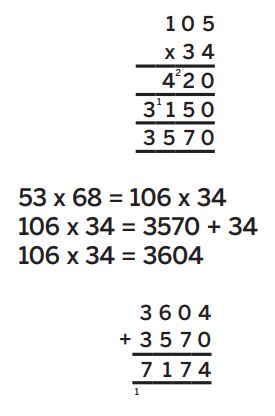
A third method uses the idea from method two but takes it a step further, using the idea that 105 x 34 + 106 x 34 is equal to 211 x 34.
Interestingly, this is the simplest multiplication to complete, with the least exchanges:
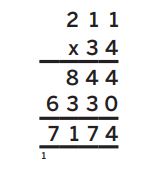
Number sense
Comparing the methods highlights the need for number sense.
Instead of diving straight into written methods, children should look at the numbers they are using and consider if they can see any links or patterns.
Sometimes there won’t be any there, but when the links are there, it can support with calculating more efficiently.
Consider how you could use this idea when answering the question below, taken from the 2018 SATs:
Ken buys 3 large boxes and 2 small boxes of chocolates. Each large box has 48 chocolates. Each small box has 24 chocolates. How many chocolates did Ken buy altogether?
In conclusion, it’s vital that we consider how we teach problem-solving strategies.
Highlighting different methods can lead to mathematical discussion and the chance to unpick the structure of a problem.
Bar modelling can represent the problem and support children with what operations they need to use to solve the problem, giving them the starting point they need.
Free KS2 SATs online 10-minute tests

These CGP 10-Minute Tests are ideal for SATs revision in Year 6. There are six tests to choose from – three for maths and three for English.
All the answers are explained at the end of each test, so it’s easy to spot any areas that need a little extra work.
If you’re looking for an offline solution, these SATs 10-Minute Tests downloads from Schofield and Sims cover maths and English for both KS1 and KS2.

Here’s another couple of free online 10-minute tests to try, from STP Books. There’s one focusing on KS2 grammar and punctuation and another on KS2 spelling.
KS2 SATs access arrangements
Some pupils with specific needs may need additional arrangements so they can take part in the 2024 KS2 SATs.
This could include, among other things, additional time to complete the test, the use of electronic aids or the use of rest breaks.
Read the government’s guidance for schools about access arrangements here.
How to plan ‘backwards’ for SATs

When working towards SATs with your class, it’s well worth planning things out ‘backwards’ starting with your desired outcomes…
The expectations of SATs have changed in many ways during my 16 years as a Year 6 teacher and phase leader, but the importance of preparing children for that end goal has not.
A child’s achievement at the end of KS2 is the collaborative responsibility of everyone who has crossed their learning path; but it’s the Year 6 teacher who holds the glue-stick that fixes all that learning together.
Extensive knowledge
To prepare children fully for what they’ll encounter during their SATs, Year 6 teachers need to have an extensive knowledge of the whole KS2 curriculum. It’s a misconception that SATs are based on the Year 6 curriculum alone; questions are drawn from right across KS2, which can be tricky if you haven’t taught the whole Key Stage.
This is where drawing on printed practice books and revision guides, such as The Scholastic Learning Journey (available at WHSmith), can help. In addition, knowing the history of your class and any gaps that have occurred in their coverage of this curriculum is important.
Familiarisation
Knowledge of the test structure itself is also an essential part of the SATs toolkit, and familiarisation with past papers is a must. Knowing and recreating frequently used question stems and structures, replicating them in class learning, knowing which content domains come up frequently, which areas are asked in greater percentages, and which skills and knowledge haven’t appeared recently, will all help with preparation.
There are many education consultants who analyse SAT papers year on year and publish these statistics online to support Year 6 teachers.
Reverse planning
Successful Year 6 teachers are forward thinkers. I recommend planning the year in reverse: start with the end goal, add in the SATs week and your final moderation of writing date, and then create a timeline, working backwards.
Put in past paper practice and decide when you want to start a revision period. Your timeline will then begin to appear. If you have a revision period date plotted in, then you know when you need to have completed your coverage of the curriculum.
This is where the knowledge of the curriculum and tests is essential. I used to map every week of the spring and summer term, ensuring I had time to cover the whole maths curriculum and to revise the key areas that I knew always appeared in the tests. I would then repeat this process with reading and SPaG.
TAF
In terms of writing, knowing the End of KS2 Teacher Assessment Framework will enable you to plan writing provision that allows opportunities for children to show their skills. Plotting out a range of different text types for writing (both fiction and non-fiction), with a range of different audiences and purposes is essential.
The text types chosen should allow children to write in different styles and levels of formality, and apply different grammatical skills, to ensure a wealthy portfolio of writing evidence for moderation.
Practice and apply
Any experienced Year 6 teacher will tell you that pace of learning, repetition of learning and opportunities to practice and apply learning are essential for SAT preparation; and indeed, being ‘secondary ready’.
To achieve this, a Year 6 teacher has to be clever with their time. Dual duty learning, where children can apply and practice reading, writing and maths skills within other curriculum areas, is invaluable. Likewise, using up those minutes of ‘dead time’ when, for example, assemblies or visitors are cancelled.
During the spring and summer term, I would always have a tray of revision questions that I could grab for quick practice moments, at times like these!
And a final thought: SATs may be a suite of tests (except for writing), but in every successful Year 6 classroom, assessment for learning underpins all the tools in the toolkit outlined above.
Knowing children, their learning strengths and areas for development, enables teachers to personalise the preparation journey.
Ruth Astley is a former assistant headteacher, experienced Year 6 teacher and phase leader, SLE for assessment and English and former LA moderation manager.
End-of-KS1 optional assessment

Have Year 2 SATs been scrapped?
Yes, they have been. Following a 2017 consultation, the government announced in 2022 that from the academic year 2023-2024 onwards, end-of-KS1 assessments are no longer statutory and are now optional.
The following KS1 assessments are now optional:
- teacher assessment judgements in reading, writing, maths and science
- tests in reading and maths (the grammar, punctuation and spelling test will also remain optional)
- where relevant, using the pre-key stage standards or engagement model
KS1 English
You can find KS1 past papers from 2019-2023 on the government website.
Year 2 SATs practice worksheets
Prepare pupils to show what they can do at the end of KS1, including in the optional reading paper, with these fun KS1 SATs practice packs from Plazoom.
All 25 packs are based on original model texts by children’s author Mike Davies and include fiction, non-fiction and poetry. Use them to help Year 2 pupils develop comprehension and writing skills.

Download a free version of Collins KS1 English SATs Study Book. It contains clear and accessible explanations, practice questions, review questions and mixed questions.
Also on offer from Collins are these free KS1 English flashcards, covering a range of topics including sounds and syllables, apostrophes, suffixes and common exception words.
Use these KS1 Revise & Assess resources from Plazoom to help Y2 pupils to create authentic writing outcomes linked to TAF statements.
You’ll get grammar recap sessions, short daily revision blasters and more – everything you need to ensure Year 2 learners are ready to shine at the end of KS1.

There are 31 free Year 2 worksheets to download at The Mum Educates blog. Each features a mix of questions on spelling, arithmetic, reasoning and literacy – all on one sheet.
KS1 maths
Year 2 SATs maths questions
This free KS1 Maths SATs Study Book from Collins features clear and colourful explanations of a range of topics, with lots of practice opportunities for pupils (and all answers included).
Also available from Collins are these KS1 maths flashcards covering topics such as counting forwards and backwards, more and less and place value.
If efficiency is your thing, this KS1 puzzles and problems PDF features 25 different reasoning and logic problems all in one place. It features a wide variety of questions covering costs, measurements and more.
This Reasoning and convincing at KS1 collection from NRICH features plenty of activities to improve mathematical reasoning skills, including A Bag of Marbles which gives children the chance to build on simple sorting skills and experience using logical reasoning.
Download our Year 2 maths worksheets.
SATs criticisms and controversies
SKIP TO A SECTION
2022 reading paper and 11+ crossover

In 2022, one anonymous primary teacher, argued that the SATs reading paper favoured the already advantaged, because a section had been on the 11+ paper earlier in the academic year...
At the end of 2022’s English reading SATs paper, something strange happened: as the children filed out of the hall, instead of the usual ashen-faced signs of exhaustion and stress, a good number came out positively beaming.
“We’ve done this before!” they said. “That was so easy!”
I had never seen a reaction like that to a reading SATs paper, the most gruelling of all the papers. I had just been invigilating a small group of children who either had extra time or who were prone to panic; none of them had found it easy.
I asked these jubilant pupils what they were talking about and was told a section had been on the 11+ paper they did earlier in the academic year. The very same extract from the very same book.
“I was told a section had been on the 11+ paper they did earlier in the academic year”
A Traveller in Time
The controversy revolved around the third and final piece that children had to read and answer questions on. Typically, this is both the hardest piece to read and comes at a point in the exam where pupils’ stamina is often flagging.
In this case, it was an extract from a 1939 text called A Traveller in Time by Alison Uttley.
In the extract, time seems to stand still as a girl passes by a mysterious lady in old-fashioned clothing on a staircase. There is a greeting smile between the two before the girl turns and follows her, only to find she has disappeared.
The text makes perfect sense if you are forearmed – as many of my children were – with the knowledge that the lady comes from the Elizabethan era and that we are in a time-slip story.
Without this knowledge, as was the case for most of the children who did not take the 11+, it would be easy to read this story literally and miss the symbolic value of much of the detail – particularly if they were struggling to stay on time.
Notifying the STA
When I notified the Standards and Testing Agency (STA), they argued that they ‘cannot entirely mitigate against pupils having read certain texts, or against them being used by other organisations.’
They also argued that checking the texts against other tests would be ‘impractical and expensive.’
“They argued that they ‘cannot entirely mitigate against pupils have read certain texts'”
Of course, if you choose to use a real and readily available text in the exam there is always a chance children would have encountered it before. It would therefore make sense for them to write the texts from scratch (or at least take a cursory glance at the biggest test our Y6 children had ever faced).
With no SATs in 2020 or 2021 due to Covid, the STA had three years to get these tests right and they did not succeed.
It failed at the single most important part of its job. After all, what is the point in standardised testing other than to provide a fair and impartial measure of the children’s abilities?
To allow a situation where a particular (often privileged) demographic of the cohort is at an advantage seems to be allowing, and possibly encouraging, bias.
2021 SATs cancelled

In late 2020, Matthew Kleiner-Mann, leader of of Ivy Learning Trust (@ivy_trust), argued that SATs should not be scrapped. In January 2021 the government did indeed go on to cancel 2021 SATs, recognising that the tests would be “an additional burden on schools” during Covid…
While teachers hate SATs (and parents love them), the vast majority of children simply accept them as part of school life. It’s just something they do at the end of school; a rite of passage like the class photo or school disco.
The prospect of them not being there is another example of how Covid-19 has robbed them of normality.
After a tumultuous few months, which have seen their lives being turned upside down, children are really happy to be back at school. They’re glad of their routine, of learning, of normal life.
So when I received an email asking me, along with dozens of other school leaders, to sign a petition to stop SATs going ahead in 2021, I wondered why people felt so strongly about it.
Is it about Covid-19, or simply a longstanding dislike of SATs? Because, whether you agree with SATs or not, now is not the right time for this argument.
Additional stress
First let’s look at the possible effect of SATs on the children taking them. Some leaders have argued that children may feel additional and unnecessary stress because of these tests on top of the ongoing pressures of Covid-19.
However, if children are feeling under pressure about SATs, then their schools are doing it wrong. This ‘cramming’ for tests should have been wiped out years ago.
“This ‘cramming’ for tests should have been wiped out years ago”
Some children have undoubtedly had a difficult time during lockdown and will need additional support but this should be dealt with individually, not by taking SATs away from everyone.
And fundamentally, SATs aren’t for children. They don’t – or at least they shouldn’t – care about the results.
They’re not like GCSEs or A-levels that have to go on your CV permanently. You can’t ‘fail’ your SATs and they don’t affect your future.
SATs are about understanding progress. They help parents to know if their child is doing OK, if they’re ready for secondary school and if they need extra support.
They help primary schools see how pupils are progressing and secondary schools understand where children are at.
Accountability
Schools should be accountable for the progress children have made and we need to know that result. You’re only able to allocate resources if you understand where the gaps are.
If we don’t find out whether a child is ready for secondary school or not, and what additional support they need, we’re doing them a huge disservice.
“I don’t believe SATs are a problem in themselves”
I don’t believe SATs are a problem in themselves. They give a good indication of how a child is progressing through their education, benchmarked nationally.
Level playing field
The primary concern now is that the same level of accountability will be applied to all schools, when they’ve had very different experiences during Covid-19 based on factors beyond their control. This includes the communities they serve, digital poverty, local outbreaks or lockdowns.
Because of these factors, it’s difficult to ensure a level playing field and schools may feel unfairly judged.
This absolutely needs to be acknowledged and addressed, but not by cancelling SATs altogether. Instead, there should be a deeper understanding and acceptance that not all schools have had the same experience in the 18 months leading up to the tests.
We need to know where a Y6 child is at, but the school shouldn’t be punished if pupils haven’t progressed as expected because of factors beyond their control.
SATs should go ahead, but with the proviso that future judgements of the school take into account the actual experiences of children during lockdown, what schools did to support their communities with home learning, and progress made in closing any gaps since schools have reopened fully.

Eleanor Regan, a researcher at the EDSK think tank, disagreed with Matthew Kleiner-Mann, arguing that SATs have a distorting and damaging effect on schools. She’s calling for adaptive online tests instead…
Overbearing one-off tests such as SATs inevitably encourage a narrowing of the curriculum, and school leaders and teachers are put in an impossible position.
Knowing there is only this one chance to show that pupils have met a certain standard encourages them to focus heavily on the topics being tested, which can limit curriculum time for non-tested subjects such as sport and art.
There is something wrong when the demands of the assessment system mean sacrificing a well-rounded education.
“[This] encourages [schools] to focus heavily on the topics being tested”
Pupil stress
Another problem is that one-size-fits-all assessments can affect pupil motivation. While there is mixed evidence about how stressful tests like SATs are for children, previous research has found that most young learners are indeed aware that their SATs results contribute to an ‘official’ judgement of them.
Pupils may feel they have ‘failed’ even if they have in fact made perfectly acceptable progress, which could alter their attitude towards learning.
Children should not have their self-esteem undermined by standardised exams that are somehow expected to suit pupils of all abilities.
Clearly, improvements to the current system could and should be made – but how? At education think tank EDSK we think that moving towards online ‘adaptive’ testing would be a sensible step.
What is an adaptive test?
Computer-based adaptive tests automatically adjust the difficulty of questions to match a pupil’s performance, with a correct answer leading to a harder question, and an incorrect answer leading to an easier question.
The research suggests that scrapping existing assessments such as SATs and moving to online adaptive tests for reading, numeracy, and spelling, punctuation and grammar would generate two significant improvements.
Firstly, low impact and shorter adaptive tests would help to reduce the current distorting effects of one-off exams.
Our report recommends that pupils would take adaptive tests approximately once every two years, providing regular updates on how they are performing throughout primary school, and relieving the pressures of one-off assessments.
Each test would last for around 30 minutes, with the results compiled, distributed and analysed electronically – meaning no additional burden on teachers.
“They would provide a more accurate reflection of every pupil’s performance”
The results would be shared with schools to update them on how children are performing and to support high-quality teaching and learning.
Parents would also be provided with a report at the end of Years 2, 4 and 6 showing their child’s most recent results, ensuring they have a strong understanding of progress.
Improving pupil motivation
Secondly, introducing adaptive tests may also help improve pupil motivation relative to the current one-size-fits-all assessments.
Because adaptive tests are personalised, they would provide a more accurate reflection of every pupil’s performance, rather than the standardised approach that we currently have.
This approach would also ensure that every pupil is motivated to succeed and does not become either disheartened by struggling with too many challenging questions, or bored by questions that are not challenging enough.
Does it work?
Other countries are already making use of the advantages that online adaptive testing offers. In Denmark all national tests for primary and junior secondary students are online and adaptive – and they have been since 2010.
Wales have recently replaced their paper-based national tests in numeracy and reading for learners in Y2-9 with online adaptive assessments, and Australia is making a similar move.
A successful transition is evidently possible, and given the ongoing move towards it elsewhere, it makes sense that we should also be considering it in England.

Kevin Courtney, joint general secretary of the National Education Union, looked into a report about why the SATs system should change…
BERA’s High standards, not high stakes report, published in 2021, had a clear and simple argument at its centre: to understand the performance, strengths and problems of primary education we don’t have to make every primary pupil go through the rigours of high-stakes assessment.
We don’t have to devote weeks of curriculum time to preparing for SATs. We don’t have to endure, against all our better instincts, the narrowing of children’s learning experience.
In place of universal testing there is a better way.
“We don’t have to devote weeks of curriculum time to preparing for SATs”
Weighted national sample
Learning from European experience, and rising above the dogmatism of English policy-makers, BERA proposed testing a weighted national sample of pupils in a broader range of topics.
This would provide a year-on-year understanding of the strengths and weaknesses of the system.
Linked to surveys of pupil, teacher and parent experience, it would provide what BERA calls a ‘richer exploration of educational processes and outcomes than the current system allows’.
New ‘assessment instruments including but going beyond literacy and numeracy tests and their current narrow remits’ would focus on broader issues on pupils’ development.
There would be surveys, for instance, to pick up topics such as cyberbullying, mental health and the impact of Covid-19.
Whatever the focus, the aim would be to understand in depth the learning and experience of pupils – and the responses of their schools.
End of SATs?
Schools would use data from the national sample to reflect on their own experiences and compare themselves with their peers.
Sample items from a national bank of assessment instruments would enable schools to provide helpful information for parents about their child’s achievements. SATs would be phased out.
BERA seeks to spark the hope that education can become a site where professional engagement and knowledge can be rekindled.
2017 reading SATs – were they really easier?

Penny Slater, deputy lead adviser for Herts for Learning, ponders what makes a reading task more accessible, after it was generally agreed that 2017’s reading paper was easier than 2016’s…
Lets begin by comparing the challenge of the 2016 and 2017 KS2 reading papers.
The first text offering in 2017 was Gaby to the Rescue. My analysis would suggest that, in many respects, this was actually more challenging than the equivalent 2016 offering, The Lost Queen.
For a start, it was considerably longer, thus demanding more stamina from our young readers. The 2017 text also boasted the longest sentence, outdoing its 2016 counterpart by 13 words.
I would argue, too, that the grammatical complexity of the sentences was heightened in 2017, with there being a lower proportion of single clause sentences in Gaby to the Rescue, compared to The Lost Queen.
Greater variety
The challenge continues: in 2017 there was a greater variety of verb forms; a greater use of pronouns and a considerably greater number of multi-clause sentences. However, we all agree that it felt easier…
Part of this is surely because we knew what we were in for.
No doubt, the children who successfully navigated the 2017 test had been well prepared with a diet of top-notch, high-quality, challenging literature which, over time, had acclimatised them to the potential challenge of the texts they would be facing.
“We knew what we were in for”
Key differences
However, in addition to the fact that we may have been better prepared for the 2017 test, there were some key differences between the 2016 and 2017 papers to which we could also attribute this prevalent sense of relative ease.
These being, from my analysis, word familiarity, word complexity and familiarity of content.
Conclusion 1: Reading is easier if children are familiar with the context
The first texts from the last two papers present this point perfectly. The impression the children may have got on first perusal of the 2017 text was one of familiarity: here is a story, they may have thought, to which I can relate.
And, no doubt, when they began to get stuck into the reading material, that feeling grew for most children – cats getting stuck up trees, money worries, not wanting to ruin clothes, water fights.
All these notions are well served by the day-to-day experience of a large number of 11-year-olds.
In order to feel at home with the first text from 2016, it would have helped to have some familiarity with the following notions: garden parties, houses with large grounds (including lakes with islands), adventures without adult supervision (on a lake… with no life jackets!), family crests, aristocratic traditions.
Not your daily topics of conversation for the vast majority of children, you will most likely agree.
Conclusion 2: Reading is easier if the words are more commonplace
With this in mind, my analysis would suggest that a large proportion of the words from the first text in 2016 would have presented a significant challenge to most children.
For evidence to support this claim, we can turn to the Children’s Printed Word Database (created by Materson et al), which records the frequency of words used across a huge range of children’s literature.
This tells us that despite being avid readers of good quality, age-appropriate literature, children are unlikely to regularly (or even occasionally) encounter the words ‘shallows’, ‘monuments’ and ‘ancestors’.
Many more examples from The Lost Queen may have only fleetingly crossed their vocabulary radar.
Conversely, all of the concrete nouns listed in the first 100 words of the 2017 text are logged in the database.
Conclusion 3: Reading is easier if the words are grammatically less complex
Not only did the 2017 text contain a great deal fewer conceptually challenging words than the 2016 test, the words were structurally less problematic.
To expand on this point, there were comparatively more words in the 2016 text that contained two or more morphemes, meaning that children were battling multi-layered words in quick succession.
Each morpheme within a word – be it a prefix or suffix, or on many occasions, both – presented yet another linguistic hurdle. It would seem that these hurdles were too closely placed, or too high, for many of the 2016 cohort.
Each morpheme within a word presented yet another linguistic hurdle”
So, to summarise, the 2017 texts were challenging, no doubt about it.
However, they may have felt considerably less challenging because:
- teachers had successfully prepared children for the heightened challenge based on their knowledge and understanding of the 2016 test
- the 2017 texts were easier in several aspects, and most crucially, those aspects that matter most for allowing young readers to feel confident when tackling a reading task.
Responding to the tests in the classroom
Now that we have an inkling of where the challenge (or lack of it) lies, we can use this information constructively back in the classroom to develop our teaching.
Specifically, we need to keep up the good work in presenting children with great texts that also offer a good level of grammatical and syntactic challenge.
But, in addition, we need to seek out great texts that present children with the kind of challenge that we know makes reading trickier. I am referring to texts that…
- Place children in a different time and place – a million miles away from their own lives
- Present them with words outside of their current vocabulary range
- Use multi-syllabic and morphemically complex words for purpose and effect
Then we need to find ways to encourage our weakest readers to relish the challenge that these texts provide. Easier said than done, no doubt – but a worthy challenge, nonetheless.
2017 boycott

With a national boycott of primary school testing on the cards in 2017, Debra Kidd explored what schools should do if parents and pupils joined in…
Firstly, should a parent choose to boycott SATs, it’s really important that the school is not seen to actively encourage it.
In essence, no teacher or head should suggest to parents that they boycott.
But, if a parent, or groups of parents, choose to do so, the school will not be held accountable by Ofsted in terms of their grading.
Skewed data
Ofsted has confirmed that they will not be judged unfavourably if their data is skewed by the absence of children due to a parental boycott. However, the DfE data analysis is less forgiving.
Perhaps intentionally, the entry data does not have a code for boycotting, only for absence. And absences show up on school data. If you are a school close to floor target, this could really affect your data.
If not, you can probably take the hit and explain the context to the inspectors when they come in. What choices do you have if a parent asks to remove their child from the test?
One common response is to say that the child would have to miss two weeks of school in unauthorised absences and the parents would have to pay a fine.
This is enough to put many off, but it is wrong, and with information becoming readily available to parents, it would be a very foolish head indeed who lied about this.
Two-week window
Many have wrongly assumed that they can administer the test within the two-week window allowed for children who have been ill. This is a misconception.
You can only administer the test in these circumstances if you are certain that in the period of absence, the child has had no contact with other students and has not accessed the internet.
If they have, they cannot be entered. This means that parents do not have to keep their children off for the whole two-week period, but simply for the main test days.
For headteachers then, it makes sense to simply ask that the child is kept off with unauthorised absence for the days of the test.
“It makes sense to simply ask that the child is kept off with unauthorised absence”
One things that is clear, is that these decisions can be hard on children.
The relief of not sitting a test is often offset by worry that they are somehow letting their teachers or classmates down.
It’s vital that teachers and heads don’t pass their worry about data down onto a child whose parents, hopefully in consultation with the child, have decided to boycott.
SATs reading paper 2016

Shareen Mayers, lead primary English adviser for Sutton Improvement and Support Services, lays out the changes to the KS2 reading test in 2016…
The new KS2 reading test that took place for the first time in May 2016 focused on the English Programmes of Study that schools have been following since 2014.
There was no level 6 extension paper and instead the more challenging questions were towards the end of the test.
For many teachers, the sample 2016 reading papers were largely familiar. They had three texts – not linked by theme – which pupils had to read through before answering the questions, all in 60 minutes.
However, there were subtle differences between the old and the new tests.
1. Assessment Focuses went
Over the years, Assessment Focuses (AFs) were the bread and butter of reading or guided reading in many primary schools. However, they were replaced with a simplified focus on eight content domains (2a-h) that are primarily taken from the English Programmes of Study.
These content domains contained areas such as: ‘2b – retrieving and recording information’ and ‘2d – making inferences from the text’.
Both of these areas – which are similar to the old AF2 and AF3 – carried the most weighting on the tests.
2. There were new areas of assessment
Some of the new domains were familiar to teachers, but others were not a separate focus under the previous AFs. They included areas such as ‘giving/explaining vocabulary in context’ (2a); ‘making predictions’ (2e); and ‘making comparisons within the text’ (2h).
The latter required pupils to identify the difference between events, so it was important to encourage pupils to use key words such as ‘but’ or ‘however.
There was also an increased focus on understanding fictional texts.
3. Questions focused on the text
Previous AFs concentrated on ‘explaining and commenting on the writer’s use of language (AF5)’ and ‘identifying and commenting on the writer’s purposes and viewpoints (AF6)’.
In 2016, questions focused on the text, eg ‘Explain how the descriptions … support the idea that they were inoffensive brutes…’ (2016 sample reading paper).
“In 2016, questions focused on the text”
4. Not everything was tested
The 2016 tests placed less emphasis on text structure (eg identify sub-headings, glossary, etc.).
5. New vocabulary was important
In 2016 there was a greater focus on understanding vocabulary in context.
6. A variety of question types were still used
The following question types were included, some of which were illustrated in the sample 2016 reading paper:
- Multiple choice (eg 2c What is the main message in the story? Tick one, Q24)
- Ordering
- Matching (eg 2b Match the events to the year in which they happened, Q10)
- Labelling
- Find and copy questions (eg 2a Find and copy two more words from the poem that show that the frog was frightened, Q23)
7. Challenge was not just about level 6
The more challenging questions at the end of the paper required extended answers. Questions asked children to explain vocabulary that was unfamiliar to them, using the context to aid understanding.
“Questions asked children to explain vocabulary that was unfamiliar to them”
‘Find and copy’ questions required pupils to discover answers from within the whole page, rather than being given a specific section to explore.
The test was focused on an in-depth understanding of the more challenging KS2 curriculum; not the KS3 curriculum, like the previous level 6 test.
8. Many questions were familiar
Having a new KS2 test without any clear levels was a daunting prospect, but much of the test was recognisable from previous years.
2016 SATS leaks
Nick Gibb confirmed that 2016’s KS1 SPaG test, due to be taken by around 500,000 pupils, was pulled following accidental publication of live test papers on the government website…
The error was first spotted at a school in south-east England, one of around 700 piloting the test, after a pupil appeared to recognise the questions.
The pupil’s teacher then checked the STA website, and found that the same test had been made available as a practice paper since 26th January this year. Another teacher at the school, Charlotte Smiles, subsequently notified the STA by telephone.
Appearing on the BBC’s Today programme on 22nd April 2016, Smiles recalled that, “I was told they would look into it and get back to me. About two or three hours later they rang me back to ask me where I’d seen this paper, because they couldn’t find it – so I directed them to the right place on their website, and was met with ‘Oh, yes, that is the live test paper for 2016′.”
Inquiry
Nick Gibb then announced in a public statement that as well as ordering the Standards and Testing Agency to undertake a full investigation of the leak, he had also commissioned a full ‘Root and branch inquiry into the operations of the STA.’
The statement went on to explain that, “Schools will still need to submit a teacher assessment judgement based on pupils’ work in the classroom as has always been the case. However there will be no requirement this year for them to administer the KS1 grammar, punctuation and spelling test, or use the result as part of that assessment.
“This is a clearly regrettable incident and I am sorry for any concern it has caused teachers, parents or pupils.”
“The government was warned about this and didn’t listen”
When asked whether the error was reflective of wider problems within the exam system, ATL general secretary Dr Mary Bousted said, “The government has introduced a whole raft of new tests at primary, GCSE, AS and A-Level. Tests are highly technical constructs – you have to get them right. You have to pilot them, and you have to know that they’re valid and reliable.
“All of those things are difficult and take time. The government was warned about this and didn’t listen.”
KS2 leak
That same year, the DfE also blamed a ‘rogue marker’ for making Year 6 spelling, punctuation and grammar test answers available via the password-protected Pearson website…
The answers appeared for around four hours on a password-protected website for registered test markers, or Assessment Associates, run by the marking services provider, Pearson. There were also indications that the leak in this instance wasn’t accidental.
According to a DfE source quoted by the BBC, “While the test doesn’t appear to have leaked into the public domain and can go ahead, a rogue marker did attempt to leak the test’s contents.”
The BBC further quoted the source as blaming an “Active campaign by those people opposed to our reforms to undermine these tests.”
‘Mistakenly’ uploaded
Schools Minister Nick Gibb issued a statement on the matter before Parliament, in which he confirmed that the test was ‘mistakenly’ uploaded to the Pearson website and taken down soon after the DfE was made aware of the error by the media.
Noting that it’s standard practice for ‘key individuals’ to be granted access to assessment materials prior to the day of testing, Gibb proceeded to confirm earlier reports of a deliberate leak: “Clearly, in this system, it is essential that people in positions of trust can be relied on to act appropriately. Unfortunately, in this case, it appears that one person did not, and leaked the KS2 English grammar, punctuation and spelling test to a journalist.”
The Minister went to state that Pearson UK president, Rod Bristow, had since been tasked with investigating how the material came to be uploaded to the secure site a day early, and examining the company’s records in order to identify the individual responsible for the leak.
Gibb added, “The journalist in question took the decision not to publish the test papers and I am grateful to him for that. Although this is a serious breach – and I am determined to get to the bottom of how this error occurred – it is clear that the actions of almost every marker involved have been correct and proper, and that the integrity of the tests has not been compromised.”