Help Children Memorise Times Tables by Encouraging Them to Make Connections
How to ensure multiplication tables don't become a sticking point

- by Beth Smith

Teacher: ‘Six lots of three?’
Child: ‘18’
Teacher: ‘Three lots of six?’
Child: ‘I don’t know my sixes!’
This exchange is no doubt something that many teachers will have experienced in their classrooms. But when it comes to how to teach times tables, why are certain times tables harder to learn than others, and why do many children struggle to use the times tables they already know to help them with new learning?
Times tables are often a sticking point in maths. As teachers, we often remind children that knowing their times tables will help in many other subject areas, including fractions, area, algebra, and ratio.
Children who have not memorised these facts find problem solving a great deal more difficult due to cognitive overload. Through a mixture of rote learning and teaching for understanding, we can help these pupils and enable them to use their times tables effectively.
In the national curriculum, clear links are shown in Y3 for the four and eight times tables, but why not for the three and six times table?
In Y4, teachers need to use the children’s knowledge of times tables they already know in order to support their learning of the six times tables.
Commutativity is the first piece of the puzzle. If children fully understand the commutative law then times tables become a lot easier to memorise.
As shown by this list (below), six out of the 12 facts of the six times table can be deduced from prior knowledge just through understanding commutativity:
2 x 6 = 12 because 6 x 2 = 12
3 x 6 = 18 because 6 x 3 = 18
4 x 6 = 24 because 6 x 4 = 24
5 x 6 = 30 because 6 x 5 = 30
8 x 6 = 48 because 6 x 8 = 48
10 x 6 = 60 because 6 x 10 = 60
Don’t forget to also look for links to other times tables to make connections in children’s minds, especially between the three and six times tables.
Trending

If children compare the five and six times tables they will see a pattern.
If we know what five lots of a number is, we need one more lot to find six lots. If five lots of eight is 40, then six lots is 40 + 8. As children are working towards instant recall of the six times table, this may help them calculate more efficiently.
| 6 x 5 = 30 | 6 x 6 = 36 |
| 7 x 5 = 35 | 7 x 6 = 42 |
| 8 x 5 = 4 | 8 x 6 = 48 |
| 9 x 5 = 45 | 9 x 6 = 54 |
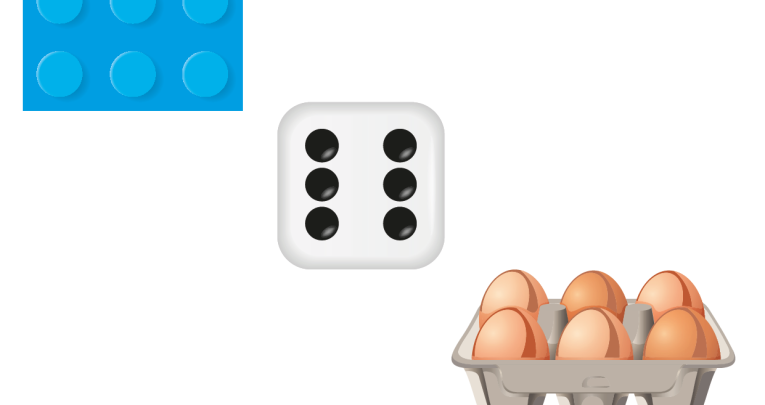
As shown with the arrays, it is important to help children understand the concept of times tables by using concrete and pictorial representations. Here are three common representations of six:

In order to build up quick recall, encourage the children to subitise with groups of six. Flash up four dice with six on each. Some children may count in sixes, others may automatically recall the fact. Through repetition, children will start to remember facts alongside a visual representation.
Memorising the six times table requires a combination of understanding and rote learning. Connections with prior learning is essential for children to be able to calculate more effectively. Daily practice of key facts should give children a much better chance of feeling confident and learning their six times table more effectively.
Beth Smith is senior primary maths specialist for White Rose Maths.
Download free resources
White Rose Maths produces free maths learning schemes for teachers which are available on TES. In its new scheme of learning, which is available to download for free no matter where your school is based, each of the times tables is given two distinct small steps: looking at the times table and related facts, and multiplying and dividing with the chosen number. Throughout the small steps, there are concrete and pictorial representations, a focus on commutativity and clear links between multiplication and division.
Download the scheme of learning at teachwire.net/teaching-resources/white-rose. Download our times tables worksheets bumper pack.