How to teach times tables – Use real-world examples

Learn how to use real-world examples so that you can make teaching times tables meaningful and engaging for all…

When it comes to maths, possibly one of the few things everyone agrees on is the importance of knowing multiplication facts. However, why we need them and how to teach times tables effectively remain hot topics debated over the ages.
Having multiplication facts and times tables knowledge as a key part of our internal ‘mathematical tool box’ is hugely important because we use them constantly in everyday life.
“For many children multiplication seems to be only something you do with numbers in mathematics sessions in school: it is not connected with any confidence to the real world.”
Understanding Mathematics for Young Children 5-9, Derek Haylock
JUMP TO A SECTION:
Big picture
Sadly, for most children (and for generations before them) the main purpose of learning these ‘times tables’ is not focused upon this meaningful and motivational application, but instead upon learning each fact on the times table grid rote-style to pass a class test, and often at speed. Which isn’t fun.
Failing to memorise and have fluent recall of these facts is cited by many adults as evidence that they are failures in mathematics, leading to a mindset that turns them off learning this wonderful subject altogether.
So, let’s agree that multiplication facts are vital skills to being a successful mathematician.
However, instead of traditional approaches using rote learning, which cause children to perform at a significantly lower level than those taught with a focus upon the ‘big picture and connections’ (Boaler, Mathematical Mindsets), let’s look at how we can all engage children with meaning and application.
We need to build strong mental connections and flexibility, working with how we know the brain learns best.
‘Why?’ and ‘how?’
Having ‘conceptual understanding’ means we know why and how something works and not just ‘how to do it’.
Traditionally, maths in the UK has focused upon procedures and memorisation. This means children fail to engage in the vital processes taking place leading to both poor performance and/or a lack of appreciation of the actual mathematics.
So, what do we mean by multiplication? The answer is not as simple as we might think. Two main structures are at work here:
Multiplying by repeated addition
This means equal-sized groups are added repeatedly.
For example: 3 + 3 + 3 = 3 x 3
Incidentally, many children will tell you 3 x 3 = 6 when they see this written down, demonstrating a lack of conceptual understanding.
Multiplying by scaling
This means a value is scaled by a specific factor.
For example: ‘The giant was four times as tall as me’, meaning ‘If I am one metre tall then 1m x 4 = 4m so the giant must be four metres tall’
Learning multiplication tables is the first of these two structures – ‘repeated addition’. But, as we can see, it’s also the knowledge on which we draw to solve problems involving scaling.
How to teach times tables meaningfully
The following tasks aim to help you learn how to teach times tables so they are meaningful and engaging for all children.
The human brain learns mathematical facts most successfully through connection and meaningful application.
Noticing and creating equal groups
Multiplication as grouping is a form of ‘quick’ counting. When we have three groups of three we could count the objects in ones – 1, 2, 3, 4 etc.
But knowing that the first group has three, that by the end of the second group we will have six, and that by the end of the third group we’ll have nine, means we only have to say three numbers (instead of nine).
This ‘quick counting’ only makes sense when children can see and connect the processes taking place.

All children need extensive experience counting in ones, and in comparing this with what happens when we only say the total of the groups we’re adding. (Three groups of three in this example.)
So they see what multiplication tables are and how they speed up our ability to count and calculate.
Watch out for ‘Mary had a little lamb….’ approaches to multiplication table learning. Teaching children to reel off a list of numbers can sound like they have multiplication knowledge: ‘Let’s count in 2s – 2, 4, 6, 8, 10…’.
“Watch out for ‘Mary had a little lamb…’ approaches to multiplication table learning”
But when we examine this more closely, they are often starting in the same place every time and have essentially just learnt to recite the equivalent of a nursery rhyme. Using and applying this knowledge flexibly then becomes almost impossible.
Using our bodies
Our bodies are a fantastic starting point for understanding and practising our times tables.
Start this activity by asking a group of children to come and stand where everyone can see them. What do we notice about equal groups of body parts? What can we see and describe?
There are multiple things. Ask: what comes in twos? Hands, feet, legs, eyes, ears? Can we see any other equal groups?

“Fingers come in groups of tens and fives,” or do they? What about thumbs? Does that mean that fingers come in groups of eight and four? How many ears do two children have? Or five children?
Learning mathematics successfully is about the process far more than the destination. We need to ensure we are praising children’s ability to explain and prove their understanding, rather than spotting facts at high speed which they may know but don’t understand (see below example).
Adult: “What’s the capital of France?”
Child: “Paris!”
Adult: “Wow, yes. That’s amazing you were so quick. Tell me about Paris and France.”
Child: “What’s ‘Paris and France’?”
‘Concrete-Pictorial-Abstract’ approach
Use interlocking cubes to represent the equal ‘human body’ groups the children notice. Legs come in groups of two so here are three groups of legs – 2, 4, 6.
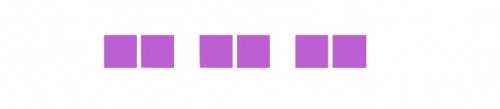
As our counting system works in base ten, use groups of alternating coloured interlocking cubes to create a number line. Reason what is happening to the totals as we as we add more equal groups.

Here we have three groups of two (representing our ‘legs’ story’) and we’re comparing them with groups of ten.
We can see that three groups of two is four less than ten. We need two more groups to equal ten.
Abstract symbols multiplication problem
Now we can talk about what we’ve built, and capture this first as language, then as symbols, to represent our understanding.
So, I can see 2 + 2 + 2 = 6
Animal leg times tables
Have you ever thought about using animals when thinking about how to teach times tables? Just think about how many different numbers of legs animals have – 2, 4, 6, 8, 10.
Some have so many it would be very difficult to count (and they don’t often stand still with that many legs!). Get children to carry out research into which animals have a particular number of legs and sort them accordingly.

Now create concrete representations.
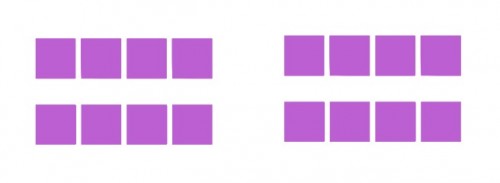
Dogs have four legs so this could be four dogs! Or lions, or giraffes, or ant eaters! 4, 8, 12, 16…
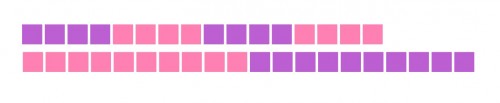
Now I can compare four groups of four on my number line made of tens. I can see that four groups of four are more than ten and less than 20. There’s space for one more group to equal 20 so five fours must be 20.
Nine times tables
Learning to count in groups of nine is considerably more challenging than counting in groups of ten. So, how can we use our understanding of counting in groups of ten to find a pattern for counting in nines?
What about 9 x 5? Well, 10 x 5 = 50. But each group is only nine, not ten. Nine is one less than ten, so there must be one missing from each group of ten.
That’s five ones, or 5 x 1, which is five. So the answer must be five less than 50, which is 45.

Eight times tables
Try this trick for working with 8s.
We know eight is two less than ten, so build an 8 x 5 model and see what you notice when you make it up to ten with different coloured cubes, just like we did with the nine.
What about other ways of multiplying by 9? 8 x 9.
Well, I know that 8 x 10 = 80, and if I’ve only got nine groups of eight then it’s eight less than 80 which is 72.
See if this works with 14 x 9.
Perhaps start with what you know about 14 x 10, build a model and make the model tell a story. For example: ‘I have nine bags with 14 items in each. How many items altogether?’.
The story helps us visualize the problem and see how imagining we had ten bags instead of nine would help us calculate.

Seven times tables
Imagine I am wondering how many days there are in six weeks. That would be seven days x 6, so I will need either my seven or six times table (because 7 x 6 = 6 x 7).
Let’s build it out of cubes. We’ll use an array:
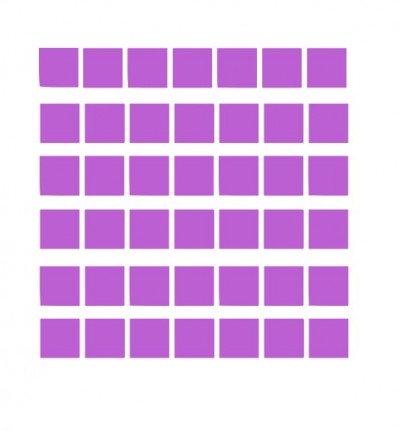
Here are six groups of seven. I don’t know how to count in 7s yet, but what can I see in the arrays? Can I see any facts that I already know or are easier to calculate?
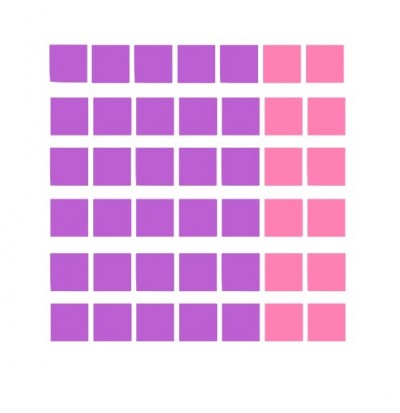
Ah, now I can see the five and two inside seven. So I could calculate 5 x 5 and then 2 x 5.
Array hunts
The equal grouping of multiplication facts is most easily seen and understood when we create arrays. We’ve used arrays already by creating equal rows and columns of cubes.
Arrays allow us to see multiplication facts easily. They allow us to partition facts into parts so we can see that 6 x 4 can be calculated as ‘double six plus double six’ or ‘5 x 9’ as ‘(5 x 10) – 5’.
“Arrays allow us to see multiplication facts easily”
Arrays in real life
Arrays exist everywhere in real life when we begin to look around us. They are a fantastic starting point for noticing and describing multiplication (and therefore division) facts.

They can also prompt some much higher-level challenge questions such as: ‘If we know the area of one of these ceiling tiles, how could we use this to calculate the area of the whole ceiling? Do we need to count every single tile?’
Download maths in real life worksheets for KS1/LKS2.

Array examples
- Drawer units
- Egg boxes
- Old windows
- Ceiling tiles
- Floor tiles
- Wire fencing
- Pictures displayed on a wall
- Chairs in lines on the hall
- Marching soldiers
- Cups arranged for coffee for a large group
- Multi-packs of water, yoghurt, cakes etc
- Chocolate chunks in a bar
- People sitting in a theatre, stadium etc
- Patterns on fabric and wrapping paper
To find out more about Karen Wilding Education and its services head to karenwildingeducation.co.uk. Download our times tables worksheets bumper pack.