Holy Trinity – Teach Your Class The Three Aims Of Primary Maths Simultaneously

Mike Askew serves up some clever activities that will let your class practise all the major mathematical skills at the same time…

- by Mike Askew

The three aims of the new primary mathematics curriculum are by now, I hope, familiar to most readers – developing fluency in mathematical skills and procedures; becoming confident problem solvers; and engaging in mathematical reasoning. There is, however, a worrying trend emerging: the view that these aims are sequential – that fluency needs to be developed before pupils can start to solve problems, and only then should they get to engage in mathematical reasoning. At a recent conference, Jane Jones, chief HMI for mathematics, commented that Ofsted inspectors were reporting seeing lessons built around this three-part progression, with only those pupils completing the fluency tasks getting to experience problem solving, and only the few who finished these problems being challenged to reason. In Jane’s words, this leads to differentiation by speed of working. If we are serious about pupils mastering mathematics, then every learner has to get opportunities to engage in all three of these aims. And there is nothing in the research on teaching and learning to suggest that fluency is a precursor to problems solving, which in turn leads to reasoning.
The evidence is that these are three linked, but largely independent, ways of being mathematical. Being good at calculating is no predictor of a child’s ability to reason. If we accept that there is no single expected way to solve a genuine problem, then many learners who are not confident calculators can be proficient at problem solving. One way to bring fluency, problem solving and reasoning together is to look for classroom tasks that will engage pupils in activities that involve both of these disciplines, while at the same time providing them with practice in fluencies.
Here I look at four such tasks – ‘Trigons’, ‘Double, Half or Stick?’, ‘Diffi’ and ‘Fab Five’. Although these are presented here in a rough order of difficultly, they can all be adapted to be made easier or harder, and so be used across a range of ages.
1. Trigons


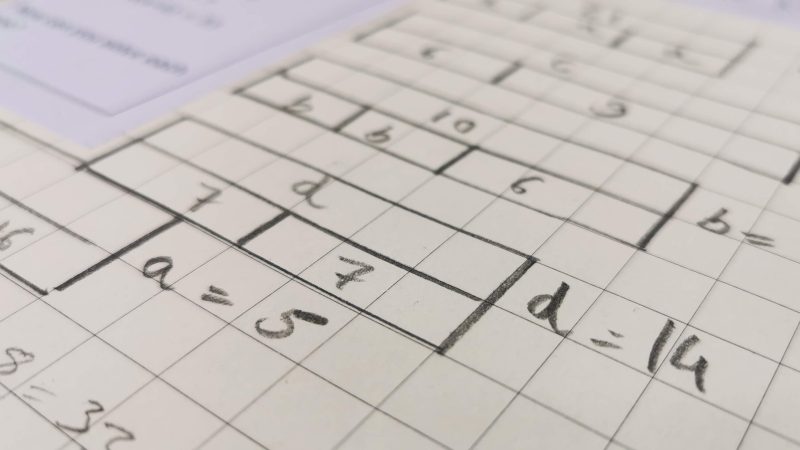
This version of the classic ‘arithmagons’ challenge makes it accessible to younger learners. Provide pupils with copies of the image in Figure 1 – they need to be large enough to put counters or cubes in each of the three sections of the triangle. Start pupils off with, say, six counters. They put some counters in each section of the triangle and write the sum of adjacent sections in the nearest square (see Figure 2). What happens if children arrange the six counters differently? Do they get different numbers in the squares? What is the largest number they can get in a square? (The class will need to agree on whether it is permissible to put no counters in a section.) Once they are familiar with the set up of the task, challenges that can be offered to pupils include:
• Given the numbers in two of the squares, and the number of counters in one section, can they figure out how many counters need to go in the other sections, and the total for the third square?
• Given the numbers in the squares, can they figure out the numbers in the three sections?
• Exploring what happens when one counter is moved from one section to another.
• What happens when one more counter is added to each section?
If pupils are confident in finding the total of the numbers in the three squares, then they can explore arranging the six counters to make this grand total as large as possible or as small as possible.
Older or more confident pupils can work with larger numbers and again, this can be extended to include fractions and decimals. Through the activity, pupils have to solve problems and reason about what is happening, while also getting to practise a lot of addition and subtraction.
2. Double, Halve or Stick?
Pupils work in pairs and need two dice between them. They roll both dice and use the scores to make a two-digit number that they agree on. For example, rolling 3 and 4 they might agree to make 34 (or 43). This is their target number. They roll the two dice again – scoring, say, 1 and 5. Working for the moment independently, each pupil uses these two digits to create a number that’s as close as he or she can make it to the target number. They do this by:
1. Using the scores to make a two-digit number – either 15 or 51 in this case. 2. Choosing whether to stick with that two-digit number, or to double it, or to halve it (they will need to decide whether, if halving results in a mixed number, to keep or discard the fraction).
For example, 15 could be doubled to 30, or 51 could be halved to 25 (the 1/2 discarded). Thirty (by doubling 15) gives the result closest to 34, so that would be number to choose in this instance.
Children compare their final numbers, checking they have arrived at these correctly. Each player’s score for that round is the difference between the final number they create and the target number (in the example above, a score of 4). The winner is the player with the lowest running total after, say, five rounds. Variations and challenges here include:
• Working with 1-10 dice or spinners
• Being given a set of target numbers in advance, say, 10, 20, 30, 40, 50
• Creating three-digit numbers
• Being allowed also to treble or take a third of a number
Again, trying out various possibilities, deciding on the best option and justifying their decisions are all part of mathematical reasoning. All that practising of doubling and halving is a bonus!
3. Diffi


Pupils draw a large square on plain paper. They write down four different numbers, one at each corner. In the middle of each side, they find and mark down the difference between the two numbers at the corners (see Figure 3). They join the midpoints of the sides to create an embedded square, marking the midpoints of this square and writing in the differences as before (see Figure 4). They continue joining midpoints to create smaller squares and fill in the differences: eventually a somewhat surprising result emerges – zeroes at all the midpoints (try it out!). The question then is whether or not a result of four zeroes will always emerge. Pupils usually want to try larger numbers, in the expectation that either something different will happen or it will take longer to get all zeroes. Surprisingly it turns out that larger numbers can often settle to zero more quickly, so one deepening of the activity is to challenge pupils to see if they can find numbers that create the most number of ‘layers’ of squares.
Other challenges include exploring whether the same thing happens with fractions or negative numbers. Not only are learners practising a lot of mental calculations, but also they are again reasoning and problem solving at the same time. Diffi has the further advantage of beings a relatively open task where pupils can choose the numbers they work with and have some control over the level of difficulty. You might think that if pupils can choose their own numbers they will go for easier options. But in my experience this happens less often than you might expect.
Yes, pupils often want to start with simple numbers – but an activity like Diffi is intriguing, and most learners quickly want to ‘up the ante’ to see if it will work with larger figures.
If, however, pupils persist in ‘playing safe’ then constraining the choice can raise the level of challenge. For example, you might introduce Diffi with the restriction that the four numbers all have to be within the range of 20 to 50, or bigger than 80 and so on.
4. Fab Five


Pupils construct a row of five empty boxes, and a single empty box separated off to the right (see Figure 5). They choose a starting number to put in the left hand box, then a second number that will be added to the running total in each of the boxes to the right of this, in sequence. For example, starting with 4 and adding 5 would produce the result in Figure 6. Having filled in the five boxes, they find the sum of the numbers in these squares, and write this in the square to the right. The challenge comes in giving the pupils the sum of the five squares and asking them to figure out what the starting number and the number added each time must be. So, suppose the total sum is 20, or 65? What are the five numbers in the squares? There is now a problem to solve and mathematical reasoning needs to be engaged in order to find the solution. Pupils might, for example, work systematically and look for patterns – they might create some more examples that always start with, say, 1 and vary the number added each time. Or they might keep the number added constant and vary the starting number.
Other pupils might use a trial and improvement approach, trying out different starting numbers and different adding numbers, and adjusting these to close in on the target number. There may be children who have a sense of this having something to do with averages, and who look at the patterns within the five squares.
(In the example above, consider the centre number – 14. As you move out pairways from this – 9, 19, and 4, 24 – what do you notice?) Finally, some pupils might explore this using algebra. Pupils could then explore what happens with six boxes – or a negative number to start with, or subtracting each time, or using fractions, or…
What makes activities like these attractive for me is the sense of inquiry they provoke. Rather than simply working through a series of exercises, through inquiry pupils are engaging with what mathematics is really about – problem solving and reasoning. Getting lots of practice in fluency just happens to be a by-product of working on rich, inquiry-based tasks.
Mike Askew is adjunct professor of education at Monash University, Melbourne and a freelance primary maths consultant; for more information, visit www.mikeaskew.net or follow @mikeaskew26